research project C33
modeling and simulation of composite materials
matheon



people | description | publications | outreach

people


head: Daniel Peterseim
researcher: Karoline Köhler


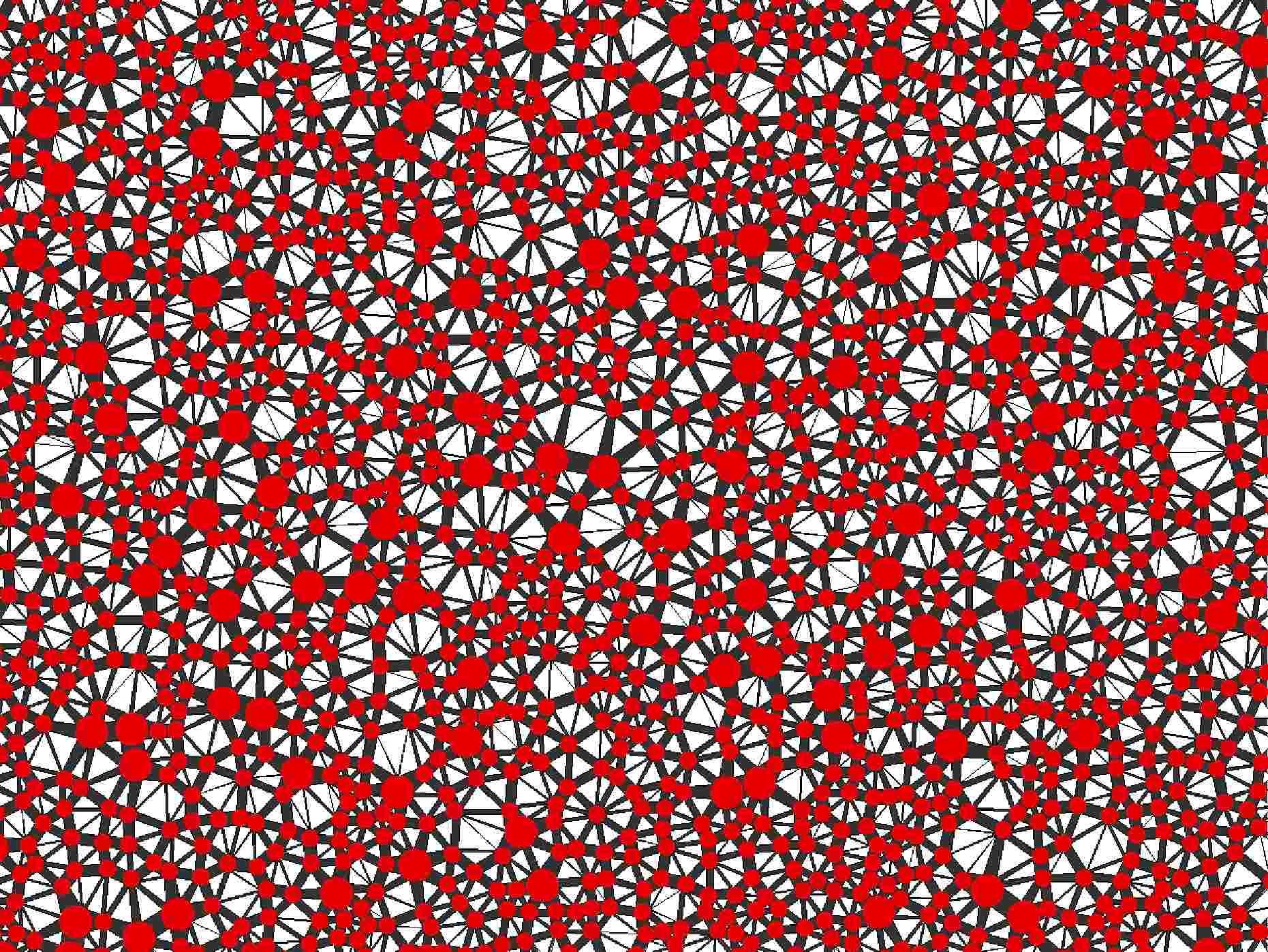
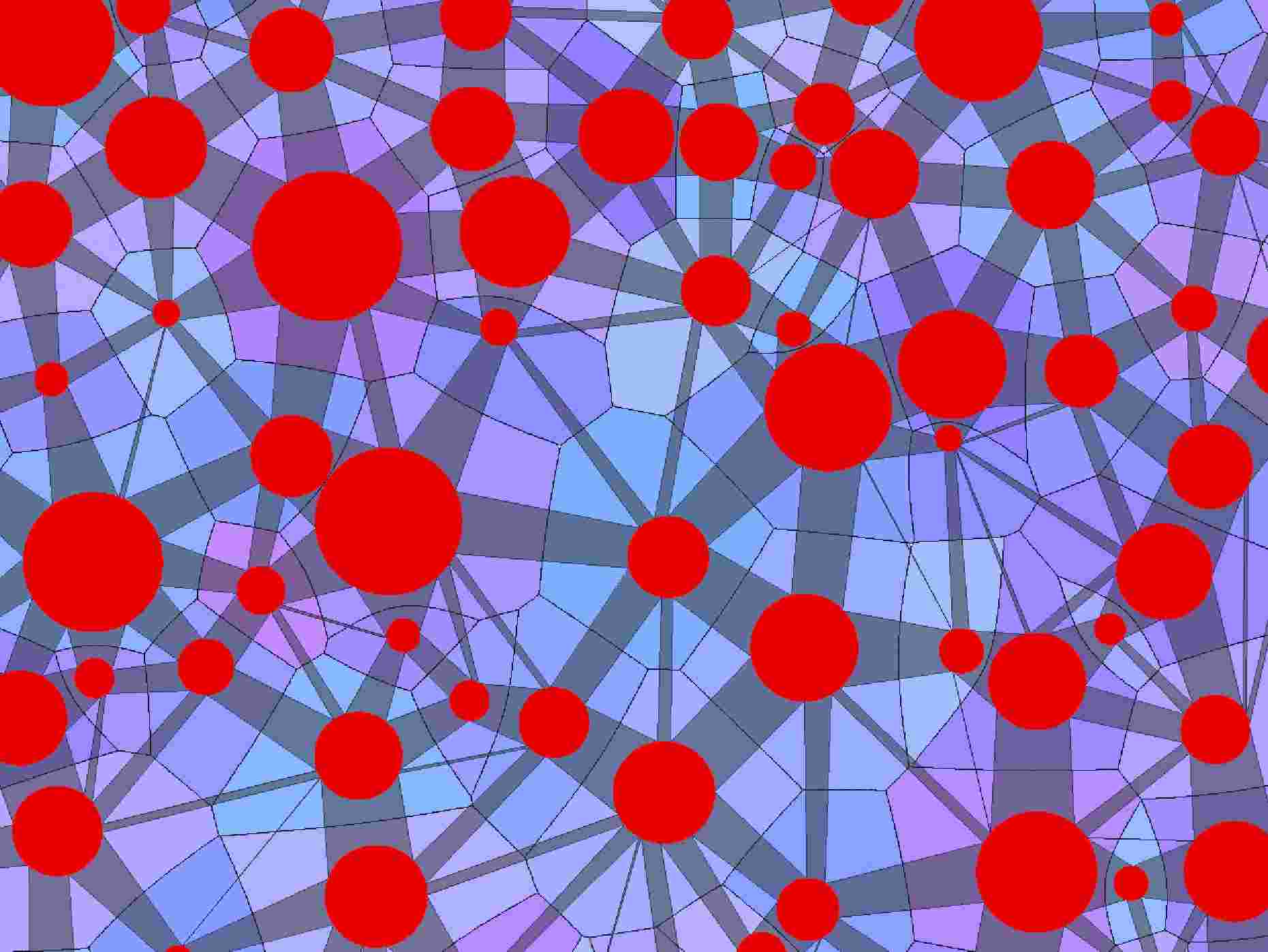
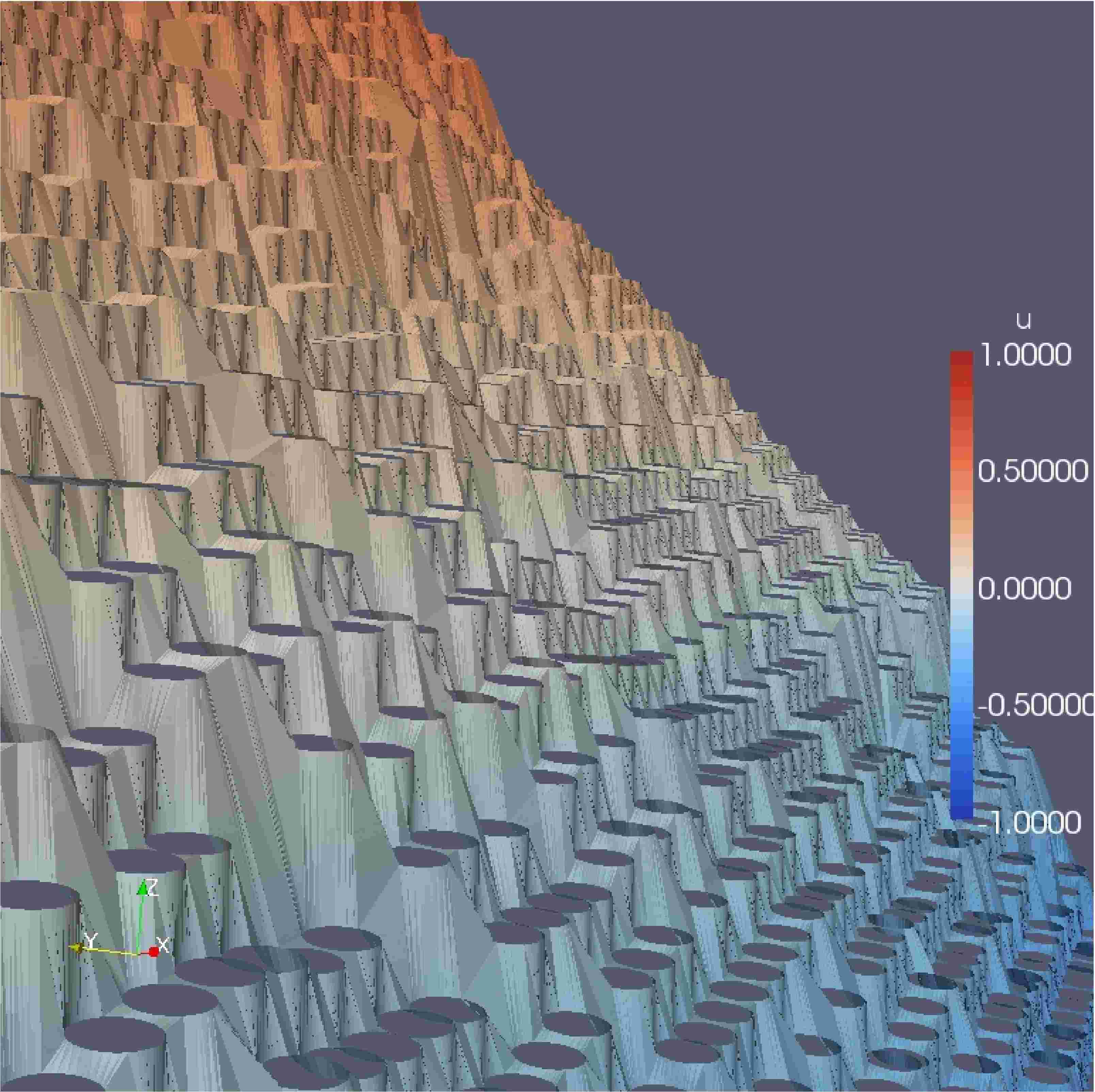
description

Composite materials are engineered materials made from two or more constituent materials with significantly different physical properties. In a typical configuration, (hard) filler particles are surrounded by a matrix of a second material which binds the filler particles together. Because the characteristics and relative volumes of both the matrix material and the various filler particles can be manipulated, these materials show an almost infinite range of physical properties. The aim of this project is to develop efficient numerical methods for the simulation of material responses. In addition, the analysis of the corresponding discrete models shall lead to a better understanding of how composites behave and how certain properties depend on the controllable variables. Both simulation and analysis provide the opportunity to develop materials with enhanced performance for the particular industrial applications.

     


publications

submitted

peer-reviewed journal articles

technical reports

conference proceedings


back to top

outreach

invited talks

  • Spectrum-preserving Multiscale Decompositions with Applications to Numerical Homogenization and Eigensolvers
    Mathematisches Forschungsinstitut Oberwolfach
    Workshop "Interplay of Theory and Numerics for Deterministic and Stochastic Homogenization"
    March 17 - 23, 2013
  • Optimal Adaptive Nonconforming FEM for the Stokes Problem
    Advances in Computational Mechanics (ACM 2013)
    Symposium "Higher-Order and DG Methods for Flow and Transport" San Diego, California, USA
    February 24-27, 2013
  • Comparison Results for Finite Element Methods
    WONAPDE 2013
    Minisymposium "Mixed Finite Element and Hybridized Discontinuous Galerkin Methods" Universidad de Concepción, Chile
    January 14-18, 2013
  • Computational Homogenization of Elliptic Multiscale Problems
    WONAPDE 2013
    Minisymposium "Stabilized and Multiscale Finite Element Methods" Universidad de Concepción, Chile
    January 14-18, 2013
  • Computational Homogenization of Elliptic Multiscale Problems
    Dissipative Spectral Theory: Operator Theory, PDEs and Numerics
    Cardiff School of Mathematics, Wales, UK
    January 7-10, 2013
  • A New Multiscale Method for (Semi-)Linear Elliptic Problems
    Numerical Analysis Seminar
    Strathclyde University Glasgow, Scotland
    November 27, 2012
  • Computational Homogenization of Multiscale Elliptic Problems
    Seminar über Partielle Differentialgleichungen und Numerik
    Institut für Mathematik, Universität Zürich, Switzerland
    October 4, 2012
  • Scale-Adapted Finite Elements for Elliptic Problems with Highly Varying, Non-Periodic Coefficients
    Minisymposium "Theory, numerics, and applications of higher-order methods"
    CMAM-5 2012, Berlin
    July 31 - August 3, 2012
  • Localization of Elliptic Multiscale Problems
    Minisympoium "MS 119 - Mathematical and computational analysis of concurrent methods for multiscale material modeling"
    WCCM 2012, Sao Paulo, Brazil
    July 8-13, 2012
  • Finite Element Computational Homogenization of Multiscale Elliptic Problems
    Kolloquium der Arbeitsgruppe Modellierung-Numerik-Differentialgleichungen, Technische Universität Berlin
    June 19, 2012
  • Comparison of Finite Element Methods for the Model Poisson Problem
    Mathematisches Forschungsinstitut Oberwolfach, Workshop "Theory and Applications of Discontinuous Galerkin Methods"
    February 19-25, 2012
  • Finite Element Discretization of Multiscale Elliptic Problems
    Mathematisches Forschungsinstitut Oberwolfach, Workshop "Advanced Computational Engeneering"
    February 12-18, 2012
  • Finite Element Discretization on Elliptic Multiscale Problems
    University of Leicester, Leicester, United Kingdom
    November 3, 2011
  • Finite Element Methods for Eliptic Problems with Heterogenous and Oscillatory Coefficients
    Maths and Applications Sussex Seminar, University of Sussex, Brighton, United Kingdom
    June 23, 2011
  • Introduction to the Finite Element Method
    International Workshop on Advances in Computational Partial Differential Equations, BITS-Pilani Goa Campus, India
    February 07 - March 05, 2011
  • Numerical Methods for Partial Differential Equations with Multiscale Data
    Berlin-Seoul Workshop, Yonsei University Seoul, Korea
    November 15-19, 2010
  • Finite element analysis of random particle composites
    Minisymposium "    Numerical Methods for Inhomogeneous Materials"
    5th Scientific Computing Seminar, CAU Kiel
    June 28-30, 2010
  • Approximating Effective Properties of Random Particle Reinforced Composites
    Minisympoium "MSP03 - Analytical and geometrical problems in continuum mechanics"
    SIMAI 2010, Universita di Cagliari
    June 21-25, 2010
  • Finite Element Methods for the Approximation of PDEs on Domains with Complicated Micro-Structures
    Young Researchers' Minisymposium "Numerical and Analytical Methods for Problems with Multiple Scales"
    GAMM 2010, Karlsruhe
    March 22-26, 2010
  • Finite Element Analysis of Composite Materials
    Seminar über Partielle Differentialgleichungen und Numerik,
    Institut für Mathematik, Universität Zürich
    December 17, 2009

talks

  • Non-conforming Finite Element Methods for the Obstacle Problem
    Session "Guaranteed Error Bounds"
    Admos 2013, Lissabon
    June 3 -5, 2013
  • Non-conforming Finite Element Methods for the Obstacle Problem
    Mafelap 2013, London
    June 11 -14, 2013
  • Non-conforming Finite Element Methods for the Obstacle Problem
    RMMM 2013, Jyväskylä
    June 3 -5, 2013

    • organized workshops

    • Computational Multiscale Methods
      Mathematisches Forschungsintitut Oberwolfach
      Organizers: Carsten Carstensen, Berlin/Seoul, Björn Engquist, Austin/Stockholm and Daniel Peterseim, Berlin
      details
      June 22-June 28, 2014
www.math.hu-berlin.de/~numa | MATHEON project C33 | last updated: 2013-16-05